In this article we will learn the formulas for calculating capacitors connected in series and parallel, and also understand regarding the various parameters associated with capacitors in electrical circuits, in conjunction with inductors.
Capacitance which denoted as C and measured in Farads is internally made up of two identical parallel plates. The various parameters associated with these two plates are:
The product of the area (A in meters) of each of the plates, the distance (d in meters) separating the plates, and the dielectric constant symbolized as ε, and measured in Farads/meter of the space separating the plates.
ε signifies the total dielectric constant and is defined as the product of the dielectric constant of free space (ε0 ) and the relative dielectric constant of the material (εr).
Remember that the units of length and area can be metric or English as long as these may be consistent with the relevant calculations.
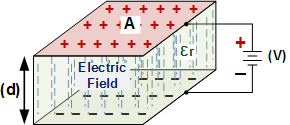
The formula for calculating capacitance with regards to its plate dimension as explained above may be witnessed in the following formula:
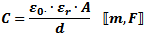
The below shown physical constants and mechanical dimensional variables may be used in equations which are discussed on this page.
The respective Units for the results of the equations may be seen inside brackets at the end of each equation; for e.g., 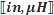 denotes lengths in inches and inductance in Henries.
denotes lengths in inches and inductance in Henries.
If units are missing or not indicated, that would signify to be consistent across all entities; i.e., all meters, all µF, etc.
C = Capacitance
L = Inductance
W = Energy
ε0 = 8.85 x 10-12 F/m (permittivity of free space)
εr = Relative permittivity (dimensionless)
µ0 = 4π x 10-7 H/m (permeability of free space)
µr = Relative permeability (dimensionless)
1 meter = 3.2808 feet <—> 1 foot
= 0.3048 meters
1 mm = 0.03937 inches <—> 1 inch
= 25.4 mm
Also note that, the dots (which should not to be mistaken with decimal points) are employed here for representing multiplication so that ambiguity can be avoided.
How to Calculate Capacitor Series Parallel Connections
Equations regarding how to connect capacitors in series and parallel can be witnessed in the following discussion. These statistics and formulas basically suggest that capacitance is actually a calculation indicating the ability of the 2 plates of the capacitor to maintain an electric charge.
Split up and separated with a dielectric (insulator), a net positive charge will be built up on a single surface area of the plate and a net negative charge will be stored on the other surface area of the plate.
For an ideal capacitor, this charge may be expected to remain stashed across the plates forever; but sadly in real life capacitors this charge progressively is shed off because of leakage currents due to the nonideal nature of the dielectric.
Series-Connected Capacitors
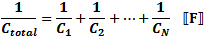

Total capacitance value of capacitors connected in series will be equal to the reciprocal of the sum of the reciprocals of each of the capacitances. The unit will be as in the original values.
Parallel-Connected Capacitors


Total capacitance value for capacitors joined in parallel will be equal to the sum of each of the capacitances, the unit of measurement being as used in the original parts
Capacitors are actually passive components applied in electronic circuits to store and retain energy as electric field. These are often used as a compliment of inductors, which also store electricity but by means of a magnetic field. A perfect capacitor will be the similar of an open circuit (incalculable ohms) for direct currents (DC), and provides an impedance (reactance) against alternating currents (AC) which varies according to the frequency of the current (or voltage). The reactance (resistance to current circulation) of a capacitor is inversely proportionate to the frequency of the signal working on it. Capacitors had been formerly known as "condensers" for a motive which goes time for the days of the Leyden Jar in which electric charges were considered to build up in plates by way of a condensation approach.
The characteristics of capacitance of resisting an alteration in voltage is taken advantage of for signals having a greater frequency component, whereas stopping signals with lower frequency elements from getting through.
A typical implementing a capacitor within an RF (radio frequency) circuit is in which there may be a DC bias voltage that must be stopped up from becoming found in a circuit but permitting the RF signal to get through. DC power supplies make use of big capacitance values in parallel together with the output terminals in order to filter out low frequency ripples as a result of rectification and/or changing waveforms.


If employed in series or parallel with an inductor, the inductor-capacitor collaboration produces a circuit that will resonates with a specific frequency which will depend on the values of each part. Within the series circuit, the impedance against current flow in the resonant frequency will be zero using ideal parts. Within the parallel circuit, impedance against current circulation will be limitless by using ideal components.
Practical capacitors created from real elements additionally present a natural capacitance whenever applied within an AC circuit.
A typical circuit simulation design is demonstrated below.

It offers typically the perfect capacitor having a parallel resistive component ('Leakage') that will react to alternating electric current. The same DC resistive component ('ESR') is within series with the ideal capacitor and an equal series inductive element ('ESL') exists as a result of metal leads (in case existing) and qualities of the plate areas. This particular inductance, along with the capacitance, produces a resonant frequency after which the capacitor appears like a natural resistance.
As the functional frequency is enhanced beyond resonance (aka self-resonant frequency, or SRF), the circuit reacts as an inductance instead of a capacitance. Consequently, cautious consideration of the SRF is necessary while picking capacitors. SPICE-type simulators utilize this or a much more advanced design to accomplish a lot more accurate calculations over the broad variety of frequencies.
Leave a Reply